DrSclafani answers some questions

[drsclafani]

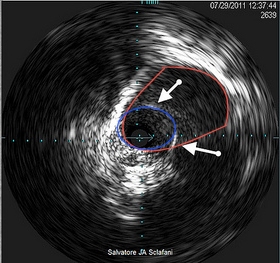
If a vein is shaped like an oval it has two diameters. Which one are you using for the balloon size, or is it somewhere in between? I suppose there is a mathematical way of calculating the circumference or the radius it would have if it were round. Are you getting that finicky about it? If so, good!

which one indeed. NEITHER

Look at the following image



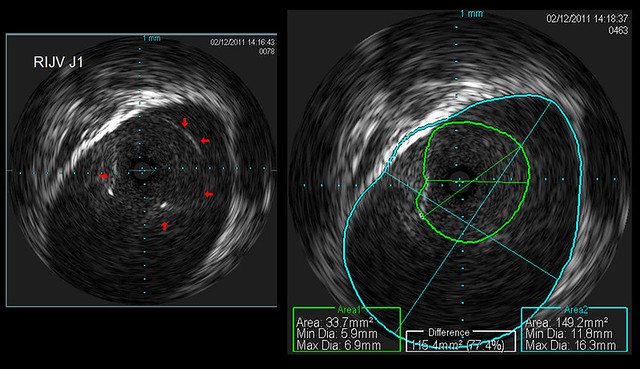
There are two colored circles. One (blue) represents the inner wall of the vein. The other (green) represents a stenotic opening of a valve. Measurements are made to the right of the vein wall (Area 2). the computer measures the shortest (11.8 mm) and the longest (16.3 mm) diameters. Above these measurements is the actual cross sectional area (149.2 mm2)

The stenotic valve had diameters of 5.9mm by 6.9mm, with a cross sectional area of 33.7 square millimeters.

So we can say that the percentage stenosis is 77%.

With the cross sectional area of the vein now known, we can calculate readily a balloon whose cross sectional area would equal or exceed that of our vein

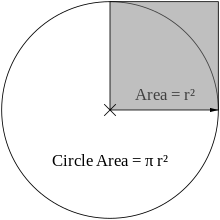
All noncompliant balloons will inflate as a circular cylinder with a cross sectional area that can be calculated by the formula cross sectional area of a circle equals Pi times the square of the radius. . It doesnt matter what the dimensions of the vein are, it will conform to the noncompliant balloon if the balloon is larger than the vein.



So calculation of each balloon based upon the known diameters give us the following table



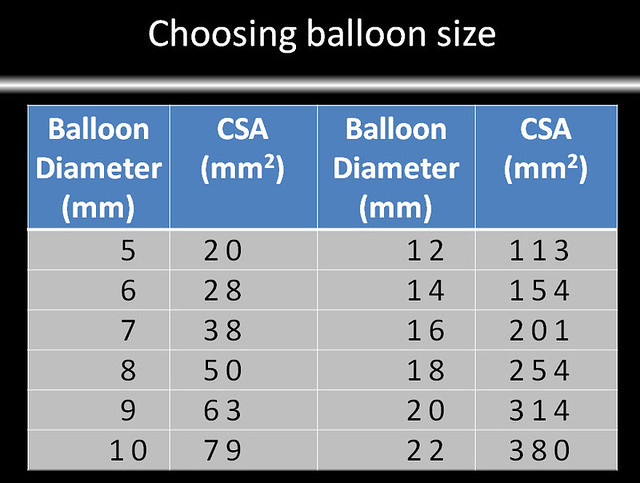
So I would choose not a 14mm balloon but would start with a 16mm balloon which has a cross sectional area about 33% larger. If the stenosis does not resolve by inflation to high pressure, I would increase the size of the balloon tgo 18mm.


the challenge will be to determine what percentage difference between the CSA of the vein and CSA of the balloon is too much or too little.

[HappyPoet]

Cece, I understand now that the arrow is pointing to the entire vertical, squiggly, speckled line; I learned from an earlier case that this type of line usually represents a valve flap (and maybe some septums).

My mistake was in thinking that the arrow was pointing to a unique signal on the squiggly, speckled line close to the green line... I kept repeatedly squinting, over and over again, trying to find a special squiggle/speckle that differentiated itself from the other squiggles/speckles, lol. Finally, I threw up my hands in frustration and asked.

Your explanation is really terrific. I didn't know the reason why a valve flap signal appears is that the flap has a thick enough edge for the echo to bounce off of -- which makes perfect sense. Much thanks! DrS, your explanation is terrific, too. Much thanks!

[Cece]

All noncompliant balloons will inflate as a circular cylinder with a cross sectional area that can be calculated by the formula cross sectional area of a circle equals Pi times the square of the radius. . It doesnt matter what the dimensions of the vein are, it will conform to the noncompliant balloon if the balloon is larger than the vein.

I found this interesting when you mentioned it before: even though the vein is oval, you don't need a correspondingly oval balloon, which would be a hassle. Veins are flexible and will take the shape of the circle of the balloon when it is inflated.

Great explanation. Lots of math. And I recognise that vein....

the challenge will be to determine what percentage difference between the CSA of the vein and CSA of the balloon is too much or too little.

If you have not been seeing thrombosis since taking on your current methods, then the percentage difference you're using is not likely to be too much but could be too little?

Earlier you said, to one poster particularly, that the days of pioneering are not yet done. I think it will be a relief once they are.

[NZer1]

Hi all, the question I am pondering is about the cases of the vein damage.

Has there been any indication as to what is the reason for thrombosis or clotting off?

Is it the remains of a valve or damage done to the vein wall?

The next line of thinking has been about the ability of the IR to "see" an area of interest then investigate. When I commit to being treated I want to know that no stone is left unturned. In my opinion if IVIS is not used there is too much likely hood of missing the unseen by the naked eye. With the cost and travel I would hope that all IR's are taking time to investigate rather than assume. To many times I am hearing that during re-treatment problems are being found that were not "seen' during the first round, yet the would have existed. Not meaning to be critical of the IR, rather the amount of knowledge at the time.

Dr. do you think you are more thorough now when you are treating than after you had treated your fist 50 patients?

Is it possible that people had problems overlooked because it was assumed that the easily identified problem would be the only problem?

I am impressed with the findings because of IVIS use. Would it be fair to say treatment without IVIS use is going to be second rate?

Many of my thoughts stem from having a friend who is wanting to book a third treatment and I am getting to a point in time when I to will be traveling.
Never been to NY, yet!

[CureOrBust]

If you have not been seeing thrombosis since taking on your current methods, then the percentage difference you're using is not likely to be too much but could be too little?

I would hazard a guess that Dr Sclafani made the whole "do no harm" oath when he got his license.

[Cece]

Would it be fair to say treatment without IVIS use is going to be second rate?

I don't think he can answer this without derogating his colleagues' techniques.

You can also ask:
* Is entering through the femoral instead of the saphenous vein second rate, because of risk to the femoral if multiple procedures ensue?
* Is not looking at the iliac and renal veins second rate?
* Even if you look at the iliac and the renal veins, is ballooning instead of stenting the iliac and renal veins, when compression means it'll return to how it was, second rate?
* Is not interogating the dural sinuses second rate, since issues can be missed and you have not followed the jugular to its point of origin?
* Is using anesthesia second rate, because you do not have the patient's participation ("hold your breath")?
* Is not imaging the ascending lumbar veins second rate?
* Does not having at least a decade of experience make an IR second rate, if there is a lack of some of the intuitive knowledge gained through experience?
* Is not using a sheath through the heart (?) second rate?
* Is not using a true anticoagulant second rate?

These are all technique differences. I like the thought Dr. Sclafani has put into his choices.

[drsclafani]

All noncompliant balloons will inflate as a circular cylinder with a cross sectional area that can be calculated by the formula cross sectional area of a circle equals Pi times the square of the radius. . It doesnt matter what the dimensions of the vein are, it will conform to the noncompliant balloon if the balloon is larger than the vein.

I found this interesting when you mentioned it before: even though the vein is oval, you don't need a correspondingly oval balloon, which would be a hassle. Veins are flexible and will take the shape of the circle of the balloon when it is inflated.

Great explanation. Lots of math. And I recognise that vein....

the challenge will be to determine what percentage difference between the CSA of the vein and CSA of the balloon is too much or too little.

If you have not been seeing thrombosis since taking on your current methods, then the percentage difference you're using is not likely to be too much but could be too little?

correction, i have seen one. I dont think it was caused by overdilation but rather to other factors. It did require another intervention to get a very significant improvement.

But generally i might be overdilating without creating a complication. But is that the goal? I think not. We should be looking for a "perfect" dilatation?

Earlier you said, to one poster particularly, that the days of pioneering are not yet done. I think it will be a relief once they are.

not sure when pioneering will end in this. There are so many questions to answer.

[drsclafani]

Hi all, the question I am pondering is about the cases of the vein damage.

Has there been any indication as to what is the reason for thrombosis or clotting off?

Is it the remains of a valve or damage done to the vein wall?

We have not discussed causes of thrombosis in a long time
1. inadequate anticoagulation
2. stenting
3. dissection of the vein
4. excessive trauma to the vein.

I personally think it is unlikely that tearing the valves will result in thrombosis.

Of course detecting the cause of thrombosis discovered weeks or months later is about impossible.

The next line of thinking has been about the ability of the IR to "see" an area of interest then investigate. When I commit to being treated I want to know that no stone is left unturned. In my opinion if IVIS is not used there is too much likely hood of missing the unseen by the naked eye. With the cost and travel I would hope that all IR's are taking time to investigate rather than assume. To many times I am hearing that during re-treatment problems are being found that were not "seen' during the first round, yet the would have existed. Not meaning to be critical of the IR, rather the amount of knowledge at the time.

Dr. do you think you are more thorough now when you are treating than after you had treated your fist 50 patients?

let me count the ways
1. using left saphenous vein instead of left femoral vein, instead of right femoral vein
2. using ivus better. Not just using it but recognizing problems.
3. better estimating significant stenosis
4. interrogating the dural sinuses
5. always look at the left renal vein
6. imaging the azygous vein in inspiration instead of expiration
7. using ivus to confirm questionable renal vein compression
8. use of anticoagulation
9. increased reluctance to try to dilate hypoplastic veins

i am sure cece will fill in what i have forgotten

Is it possible that people had problems overlooked because it was assumed that the easily identified problem would be the only problem?

people see what they are prepared to see.

I am impressed with the findings because of IVIS use. Would it be fair to say treatment without IVIS use is going to be second rate?

It would be fair to say that i would prefer to perform IVUS in all of my patients.

Many of my thoughts stem from having a friend who is wanting to book a third treatment and I am getting to a point in time when I to will be traveling.
Never been to NY, yet!

new york, ny Its a helluva town, broadway's up and the battery's down

[drsclafani]

Would it be fair to say treatment without IVIS use is going to be second rate?

I don't think he can answer this without derogating his colleagues' techniques.

You can also ask:
* Is entering through the femoral instead of the saphenous vein second rate, because of risk to the femoral if multiple procedures ensue?
* Is not looking at the iliac and renal veins second rate?
* Even if you look at the iliac and the renal veins, is ballooning instead of stenting the iliac and renal veins, when compression means it'll return to how it was, second rate?
* Is not interogating the dural sinuses second rate, since issues can be missed and you have not followed the jugular to its point of origin?
* Is using anesthesia second rate, because you do not have the patient's participation ("hold your breath")?
* Is not imaging the ascending lumbar veins second rate?
* Does not having at least a decade of experience make an IR second rate, if there is a lack of some of the intuitive knowledge gained through experience?
* Is not using a sheath through the heart (?) second rate?
* Is not using a true anticoagulant second rate?

These are all technique differences. I like the thought Dr. Sclafani has put into his choices.

cece
you are very kind. But the real answer to whether care is first or second class will depend upon outcome, not any outcome but quality outcome data, intimately acquired in detail from patient and neurologist collaborators

[Cece]

In the drsclafani thread, we can definitely cheerlead for drsclafani. ;)

I wonder how long it will be until we have that quality outcome data.

I think you would agree that your techniques are strong contenders, or they wouldn't be your techniques.

Maybe it is time for another case to be posted? It has been at least a few days. Or should we talk more about the balloon-CSA post?

[NZer1]

Thanks Cece, Thanks Dr.S.
I knew the questions would be challenging to answer, I had to ask though.
Have applied for passport renewal!
Regards Nigel

[NZer1]

Dr, I have just woken up from my mid day recharge and had brain activity whilst asleep. I believe that the changes in flow that you are creating will be difficult to back track where in the brain is gaining the symptomatic improvements.
In my understanding it will be at capillary level that the changes are happening. The flow dynamics around the brain will be changed and take time to settle after the treatments you achieve IMO. My understanding of the flow is that the position of the out lets to flow is not specific to the region of the brain having flow improvements locally. The brain being a container more than system of chambers and piping when discussing out flow volumes.

I read somewhere that blood pressure is determined in the brain stem and I now cannot find where I found that. I am aware I have BP that is progressively lowering over years as are my MS symptoms and mostly 'fatigue' increases. If my BP lowers so will my CSF flow decrease. One being dependent on the other. I have or had C2 spine lesion and other brain lesions. If the CSF flow is compromised so is the CSF function, IMO. Waste products and low oxygen and glucose levels in the CSF will be depleting more in the higher resistance regions of the brain and areas dependent on more flow. (PPMS)

What veins drain the brain stem in general?

The next question is the veins that drain the Thalamas/ Hypothalamus region? This is the area of the brain that many of our symptoms have an inter joining connection of nerve axons (like a post office junction building). I remember Dr. Zivardinov saying that the venous flow is often compromised at the thalamus and MS lesions form in the area. Something that got his attention as well.

Are there specific veins that drain the Thalamus/ Hypothalamus?

Do you know if the Hubbard team have any insights with their connecting MRI testing to CCSVI treatments? I wondered if the re-stenosis that Devin Hubbard suffered gave any insight, assuming he would have been studied thoroughly?

Regards Nigel

[NHE]

How would you describe the cross sectional patency of this vein? In the image below, would it be the area outlined in blue or the area outlined in red? Or, perhaps some other area?

cross sectional area in red divided by cross sectional area in blue equals percentage of stenosis. The red circle is the lumen of the vein that is continuous with the vein above from which blood flows. The valve is narrowed within the larger vein, like a funnel. that is why you can see the vein fully and see the narrowed valve within it, but the narrowed area becomes the bottleneck. is that clear?

Yes. Thanks. I think I've got it now. % stenosis = 1-[(stenosed csa)/(open csa)]*100 or 1-(blue area/red area)*100 in this example. By the way, what is the thickness of the image slice (or how much depth is shown by the image)?

In addition, in the longitudinal compilation image, would it be correct to interpret the white line which seems to appear in the middle of the vein as a membrane splitting the vein in two? Or, is this the inner intimal wall of the vein in this section with the vein lumen to the left of the white echogenic line? If neither is correct, how do you interpret this line?

The probe is in the middle. sound reflects off the walls. on the left is echo off the wall of the vein. To the right first there is echo off the stenotic valve (the white line in the middle). Further to the right is echo off the opposite wall of the vein.

Is that clearer?

Yes. Thank you. Do the two spots indicated by the arrows in the first image depict two of the valve leaflets? If so, would the third leaflet then be somewhere over at about the nine o'clock position, but lost in all of the other reflections? If the two spots are valve leaflets, would we ever see the separate valve leaflets in the longitudinal compilation image?


NHE

[bruce123]

Hi Dr. S.

There are usually pros and cons to the various approaches used to treat CCSVI. For example, the size of the balloon, the inflation pressure, or the use of stents. These are all debated as to the advantages vs. the disadvantages.

My questions is regarding your approach of tearing the valves. You seem to focus on the valves as often being the source of the flow problems within the veins. I have not read or heard any doctors discuss any potential problems associated with tearing the valves. I would assume that the valves are there to serve a purpose and that after you treat them they are no longer able to serve that purpose. Are there any negative aspects to your approach of treating the valves rather than just the vein?

Thank you for your dedication to the site and to its members.

Bruce.

[Cece]

Hope any procedures this week, already happened or yet to come, all go well, with unusually good improvements, no complications, and not too long of days. And no one needs to take a cab ride to the hotel with the doctor.

Remember a year ago? Just beginning to put things in place for a possible move to private practice, frustration maybe that it was necessary, frustration at being sidelined, excited about CCSVI but still skeptical?

And two years ago? Satisfied with your career, starting to think retirement after years of long hours and extraordinary dedication, and in comes a letter from Michelle, changing everything.

Those Augusts laid the groundwork for this August, and that is something to celebrate.